#4 - Let's Play Ball (Sports and Math)
If you have a child that is interested in sports you have a natural connection to math. It's impossible to watch a game on TV (or on your phone or computer) and not notice all the stats used. Just the basic act of watching a game with your child (in person or on TV) and discussing the meaning of different stats (ERA, WAR, OBP, Batting average, slugging percentage, strike to ball ratio and so many more!!!) and how they are calculated can help a child think about math.
You can use these stats to make arguments about which player you think is the best. Often times when I attempt to talk to kids about who they think is the better player (could be football, basketball or any sport) their argument is that your guy "sucks." By understanding statistics the kids can make better arguments using data to support their opinion (This will also make the Language Arts teachers happy).
Want to amp it up a bit and still have fun? You can join a fantasy league (ESPN, Fan Duel, Draft Kings for example), play the board game Strat-O-Matic (they have games for almost every sport), or even play baseball video games. When you are in a fantasy league or playing games you can use math to justify your decisions.
#5 - Running a Lemonade Stand
A great ways for kids to make the connect to math and business is to let them run their own Lemonade stand. I let my kids have a lemonade stand a couple of times a year (once when we have a garage sale) and it's a lot of fun, but more importantly there are a ton of lessons to be learned from this activity.
I have my kids keep track of all their expenses (cups, ice, lemonade packets/lemons etc.). At the end of the lemonade sale we talk about Gross Profit, expenses and Net Profit. We also talk about the cost to make a cup of lemonade (cup, ice, lemonade) and our profit per cup.
To help their bottom line I buy all their unused inventory (extra cups and unused ice). For example, if they spent $8 on cups but only used 1/4 of the cups we will use $2 as their cost for cups. But, I will discuss how this would impact their business if I didn't do this.
#6 - Become Bob the Builder - build something... anything!
One of the best things a kid can do to help their overall math skills is to just build something. A table, workbench, ramp, dog house it doesn't matter. The act of making something will help with their geometric and measuring skills.
The nice thing about building items is that it's messy, hands on and requires problem solving skills (This is nice IF your kids clean up their mess!). Usually, the first time your kid attempts to build something it will be a random collection of materials that will be nothing like they were hoping to build. This is a great chance to give a little guidance/advice about angles, measurements and sketching out a design before starting.
Please: Do NOT just turn you kids loose with power tools (drills, band saws etc.) unsupervised. You can give your kids freedom, BUT you need to make sure they are safe!
#7 How far away is the lightning (or Fireworks)?
An easy way to sneak in a little math during the 4th of July or one of the many summer storms is to calculate the distance of the firework's explosion or the lightning bolt. This gives you a chance to talk about the difference between the speed of light vs. the speed of sound (You are welcome Science teachers).
The speed of light is so fast that virtually no time elapses from when an event happens to the time you see it. The speed of sound is much slower (375 yards/1125 feet per second). For estimation purposes you can figure about 400 yards per or 1,200 feet per second. A mile is 1760 yards or 5280 feet.
When you see a lightning bolt (or firework) time the number of seconds until you hear the sound. Every second = about 400 yards. So 4 seconds is just a little less than a mile away and 5 seconds is just about a mile.
#8 - Raise a Garden
If you live in downtown Chicago this might be a bit harder, but for the rest of you this is another way to mix in a little math (and get some tasty produce!). One of the keys to a successful garden is the planning and layout. What are the dimensions of your garden? What ways could you layout your garden to maximize your space.
You can track your garden expenses (seeds, plants, fertilizer, etc) and your total harvest (we have a little weight scale we use) though out the summer. If you want to amp up your garden math you can compare your expenses to the amount of money you would have spent. Was their any kind of savings? Even if it was more expensive you can talk about the health benefits of raising you own produce.
If you want to take this to another level you can have your kids set up a small farmers stand (or go to a farmers market) to sell their produce. Just like with the Lemonade Stand, this is a good way to teach some business skills along with math skills. (As a side note: I actually did this when I was in high school. As part of a project for Future Farmers of America (no I'm not a farmer) I raised cantaloupe for 3 summers. Each morning I would harvest the muskmelon, weigh and price each one. I would put my produce in a wheelbarrow and put out by the road with a couple of signs. I would play basketball while waiting for customers to arrive. I would usually sell out of produce by lunch. I made nearly $1,000 each summer!)
#9 - Lawn Mowing Business - the side hustle
There was a time when it was common for kids to make a little pocket money by mowing the neighbors yards. Maybe it's because of all the lawn mowing services that have popped up over the years, but there seems to be fewer kids mowing lawns today. But, I would argue that the time has never been better for a teen to start a small neighborhood mowing business. Because kids are usually just using a push mower, their expenses are much less than the larger lawn mowing companies (along with not worrying about employee benefits, accountant cost, marketing, etc.). As a result, the kids can charge a lot less and still make a good profit.
Also, there are a lot of adults that remember mowing lawns when they were a kid and respect a kid today willing to work hard. And with less kids to compete with, the market is all yours.
What about the Math? Alright already!
First, your child is going to need to determine what they will charge. Is it going to be by the hour? A set rate per yard/lot? Based on square footage? Most of the lots in our neighborhood are roughly the same size so my son charges a flat rate per yard (double if the yard is actually 2 lots). How often are you going to mow? Could you charge a one time fee for the entire summer? What would you charge for this one time fee (remember: it should include a savings for the customer)?
Once you know the rate you will charge (which is you gross income) you can calculate your gross income per hour. For example, my son charges $15 dollars per yard. It takes him about 45 minutes to mow a yard. When he was calculating what he makes per hour he figured that he was making $5 for every 15 minutes. There are 4 groups of 15 minutes in an hour so he is making $20 per hour.
You can have your kids estimate their gas expenses by finding out how much gas is needed to mow a yard. For example, if it takes a gallon of gas (let's say it's $3 per gallon) to mow two yards that means it cost $1.50 in gas to mow a yard. You can find your net profit by taking you gross income and subtracting the expenses. If you want to take the to another level you can charge a rental fee for use of the mower and maybe include the cost to maintain the mower.
Other things you could do: What will you make per month from a customer? How much will you make if you mow 5 lawns during the summer. How many lawns would you need to mow to make a certain amount of money such as $1,000 or $5,000? How many hours would it take to make your goal?
#10 - Estimating the Savings and Prices when Shopping
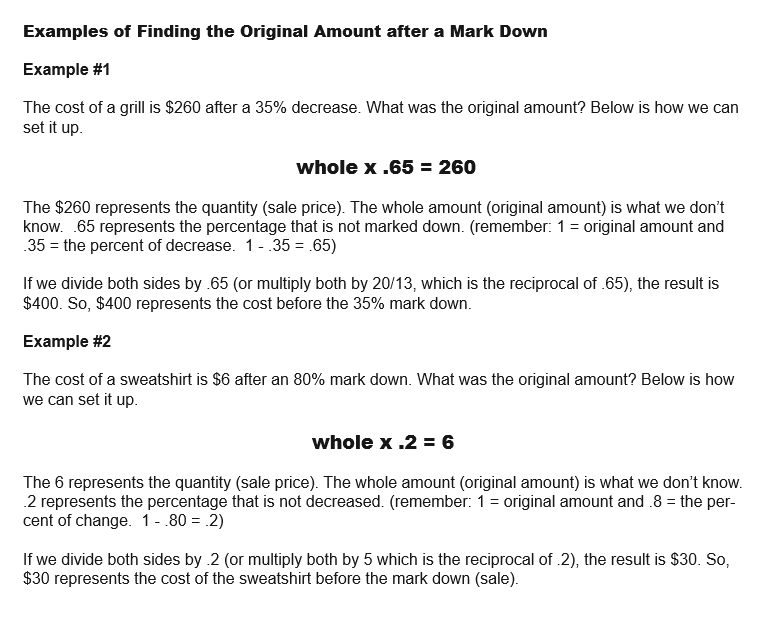
This is a great life long skill for kids to learn. This is easy to talk about in the flow of a conversation. You're shopping with you child and there is a sale! Let's say there is a 20% off sale on all lawn mowers at your local John Deere dealer. You are looking at a $2,000 mower (Apparently the lawn mowing business is working out quite well!). How much will you save? What is your cost? Many kids struggle with understanding how easy it is to find 10% of a number. In this example, 10% of $2,000 is $200. So, 20% savings would be $400 (double the 10% savings). If you are saving $400 that means that the sale price would be $1,600 (2,000 - 400 = 1,600). You can do this with any product (yes even toothpaste!).
You can then take this skill and apply this to calculating tips or taxes. For example, you are Red Lobster with your family. The bill is for $80 and you decide to leave a 15% tip. How much will you leave? If 10% = $8 then 5% = $4 so 15% = $12 tip (8 + 4 = 12).
#11 - Estimating the size of a crowd
You are at a concert, movie, baseball game or any place with a gathering of people. One simple thing to do is to estimate the size of the crowd. When you do this, don't just have your child randomly scream out a number. Have them explain how they got the number. For example, perhaps they noticed that their appears to be about 9 people in each row and since there is 100 rows the crowd is about 900 people. Maybe they count how many people are in a section and multiply that by the number of sections. You can also estimate what percent of the crowd is male or female or what percent of the crowd is rooting for a certain team.
You can do this with other things like how many Coins or M&Ms are in a jar. The correct answer is not important, the focus is on the process of trying to figure out the answer. I remember reading about how one of the questions Google used to ask possible employees was "How long would it take to wash all the windows in Seattle?" Obviously, there is no way to determine exactly how long it would take. They are just trying to see how you would TRY to solve this problem.
#12 Being Bullish on the Stock Market